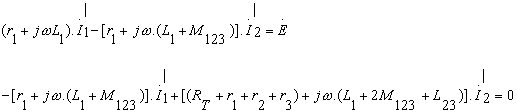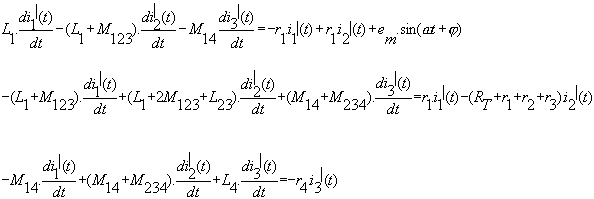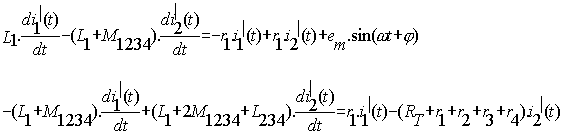STUDY OF THE TRANSIENT PROCESS LENGTH IN A STEP VOLTAGE REGULATOR
BARUDOV Emil, BARUDOV Stefan and PANOV Emil
Technical University – Varna, 9010, No.1 Studentska str.
E-mail: ugl@abv.bg
Abstract: The voltage of the individual consumers in limited power networks can be maintained within the allowed boundaries by automated step changeover of an autotransformer. Examinations of the length of the transient process as a function of the switching phase and the character of the load – R, R-L or R-C, were carried out.
Keywords: step changeover, autotransformer, regulator, model, load, switching phase
Introduction: There are electrical networks where the phase voltage varies far above the allowable limits depending on the operational modes of some of the ultimate consumers. This forces application of individual regulation of the phase voltage directly at the very consumers. The regulation can be effected by stepwise automated changeover of the autotransformer terminals. The general principles related to the solution of similar problems are discussed in [1...4].
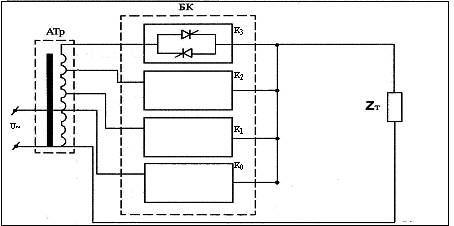
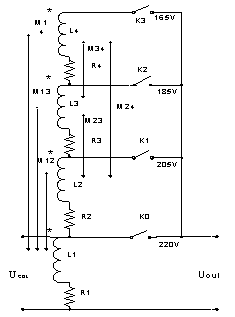
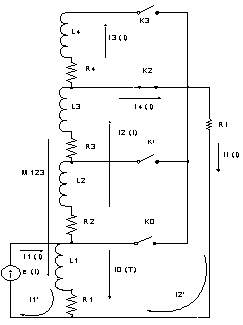
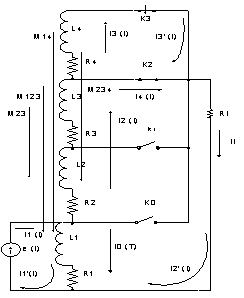
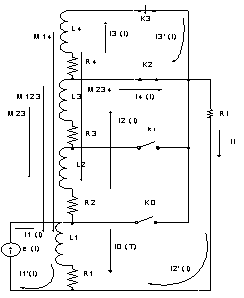
The paper presents the results of examination of the length of the transient process as a function of the switching phase of the thyristors of a respective booster element (buck converter), accounting the load character (active, active-inductive or active-capacitive). The applied mathematical model, the limitations and assumptions are indicated in the references [5]. Fig 1 presents the block-diagram of a monophase step voltage regulator, where ÁÊ is a switching block consisting of four switches K. ÀÒð is a booster transformer with terminals for changeover and ZT is a load. Figures 2, 3, 4, 5 present the equivalent circuits of the examined regulator for the three time intervals. The determination of the parameters of the equivalent circuit elements is according to [5].
The transient process should be considered within three intervals:
First interval – the voltage drops from 170V to 165V, Ê3 open, Ê2 closed, Ê1 and Ê0 open (the last but one level of the regulator is activated).
Second interval – Ê3 closes with phase delay from zero e(t), but Ê2 is not opened – there is interwinding short circuit.
Third interval – Ê3 is closed and Ê2 opens when the current i4(t) becomes zero
Fig 2 shows the voltage regulator with the existing magnetic links among the separate autotransformer sections. The description of the electrical equilibrium of the voltage regulator is made according to the mesh-current method as it leads to a minimal order differential equation systems. The examination was made for R, R-L or R-C loads with the assumption that Ê0 – Ê3 are ideal switches, which gives sufficiently accurate image of the processes.
|
|
| Fig.1 Block diagram of a monophase step voltage regulator. |
The systems of differential equations (1), (2) and (3) describe the electrical and magnetic processes in the studied time intervals. They are resolved by solving the first system (1) according to Gauss-Jordan method in the complex – frequency domain. The second and third systems (2) and (3) are solved by Euler method of numerical solution of system of differential equations.
1. Steady state mode at ![]() (first interval).
(first interval).
| (1) |
|
2. First transient process after switching of K3 (second interval).
| (2) |
|
3. Third interval – after the switching of K2.
| (3) |
|
A program AVTO1 under MATLAB was developed for determination of the response of a voltage regulator during transition from 170V to 165V, for active, active-inductive and active-capacitive loads, respectively. The program allows to define the length of the transient process for various switching phases (second interval) from 0î to 360 î , with a step of 22,5 î . The load values for the respective mode of operation are presented in Table 1.
Table 1
|
Load |
Graphic
symbols of the results for the respective load |
RT,
Ω |
LT,
H |
CT,
F |
|
R |
* |
RT=3,3846Ω |
- |
- |
|
R-L |
* |
RT=3,0675Ω |
LT=4,5531.10-3H |
- |
|
o |
RT=2,3933Ω |
LT=7,6181.10-3H |
- |
|
|
x |
RT=0,8760Ω |
LT=10,4064.10-3H |
- |
|
|
R-C |
* |
RT=3,0675Ω |
- |
CT=2,2253.10-3F |
|
o |
RT=2,3933Ω |
- |
CT=1,33.10-3F |
|
|
x |
RT=0,8760Ω |
- |
CT=9,7364.10-4F |
The following regularities were observed during the computer simulations for the studied switching of the voltage regulator:
There is a symmetry of the results in the intervals 0î ... 180î and 180î ... 360î
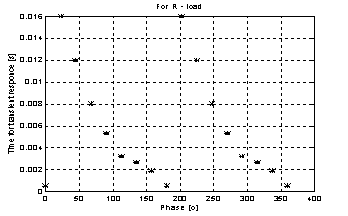
In case of active load, the length of the transient process is largest at φ=22,5î and 202,5î
and is shortest at φ=0î, 180î and 360î.
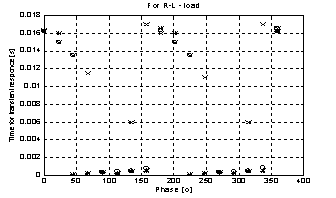
At active-inductive load, the length of the transient process is largest for the case:
“õ” – ZT=3,3846.e+j75oΩ, for almost all phases φ and is smallest for:
“*” – ZT=3,3846.e+j25oΩ , for almost all phases.
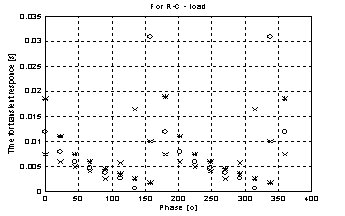
At active-capacitive load, the length of the transient process is largest for the case:
“*” – ZT=3,3846.e-j25°Ω for most of the phases and is negligibly small for
“õ” – ZT=3,3846.e-j75°Ω.
Figures 6, 7 and 8 show the graphs of the dependence of the transient process from the changes of the switching phase of the thyristors for active, active-inductive and active-capacitive loads respectively.
| |
|
| Fig.2 Equivalent diagram of the step voltage regulator |
Fig. 3 Equivalent diagram for the first interval of the transient process. |
| |
|
| Fig. 4 Equivalent diagram for the second interval.. interval of the transient process. |
Fig. 5 Equivalent diagram for the third of the transient process |
| |
|
| Fig 6 Dependence of the switching time from the change of the switching phase for active load. |
|
| |
|
| Fig. 7 Dependence of the switching time from the change of the switching phase for active-inductive load. |
|
| |
|
| Fig. 8 Dependence of the switching time from the change of the switching phase for active- capacitive load. |
|
CONCLUSIONS
The suggested model and the analytical examinations allow to define the length of the transient process depending on the switching phase and the type of the load. The experimental investigations of specific values of the phase and the load show sufficiently good correlation with the results of the computer simulation of the transient processes.
The study of the transient processes and their length is of great importance for determination of an operational mode with shortest transient processes. This is related to the regimes with most reduced loads to the switching elements and the regulator.
The suggested method can be used for calculation of booster step regulators (buck converters) for various power and operational modes. This gives rise to the idea for development of a specialized logic controller, which by tracking of the character of the load could allow switching only in the most favorable time periods, determined by the minimal risk of overloading or breakdown of the elements.
REFERENCES
[1] Minchev Ì., Penchev P. Contactless Apparatuses, Technika 1976 (in Bulgarian)
[2] Porudominskiy V. Switching Devices for Transformers under Load, Energia 1974 (in Russian)
[3] Lipkowskiy Ê. Transformer-Switching Actuator Structures of the Alternating Current Converters, Naukova Dumka 1983 (in Russian)
[4] Barudov St., Dimitrov D, Barudov Em. Functional Performance of Transformers in a Contactless Voltage Stabilizer SIELA 2001 (in Bulgarian)
[5] Barudov Em., Barudov St., Panov Em. Study of the Length of the Transient Process in a Step Voltage Regulator. Annual Scientific Session 2003 of the Technical University Varna